NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2

  

NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2

Question 1.
Find the zeroes of the following quadratic polynomials and verify the relationship between the zeroes and the coefficients.
(i) x2 – 2x – 8
(ii) 4s2 – 4s + 1
(iii) 6x2 – 3 – 7x
(iv) 4u2 + 8u
(v) t2 -15
(vi) 3x2 – x – 4
Solution: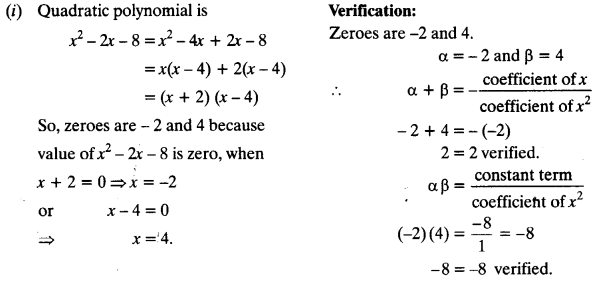

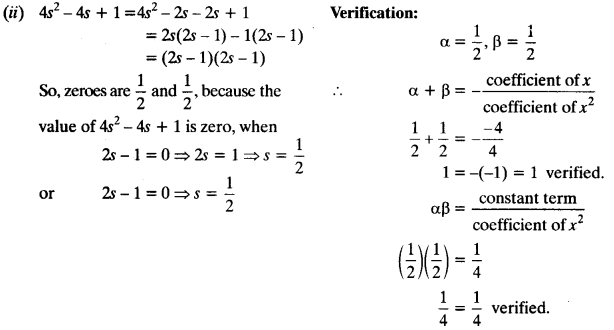
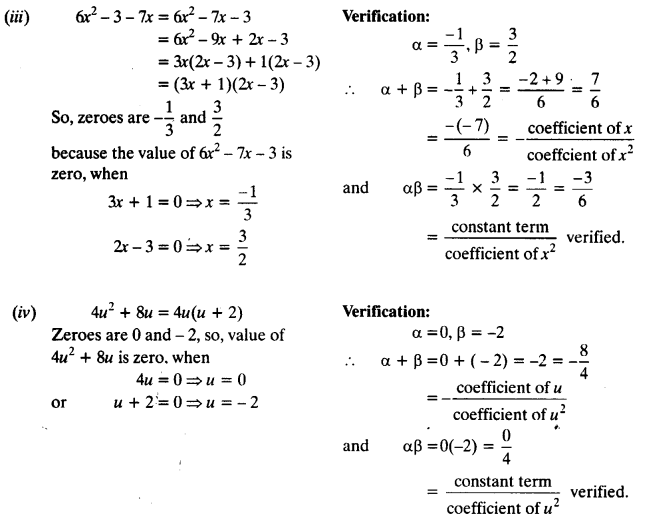
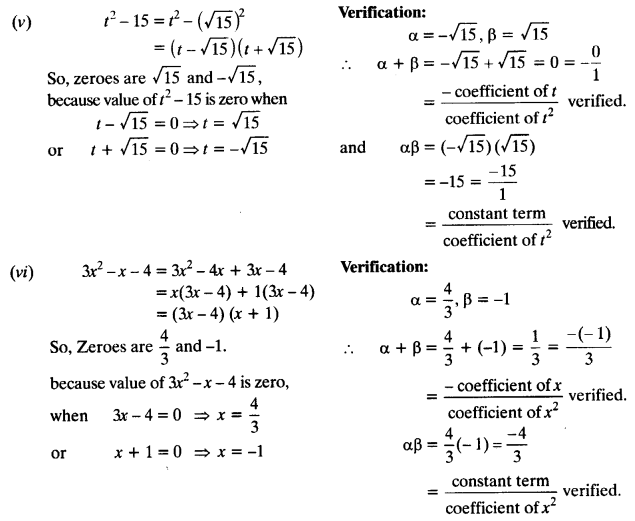
Question 2.
Find a quadratic polynomial each with the given numbers as the sum and product of its zeroes respectively.

Solution:
(i) Zeroes of polynomial are not given, sum of zeroes = 14 and product of zeroes = -1
If ax2 + bx + c is a quadratic polynomial, then
α + β = sum of zeroes = −ba = 14 and αβ = product of zeroes = ca = -1
Quadratic polynomial is ax2 + bx + c
Let a = k, ∴ b = −k4 and c = -k
Putting these values, we get

For different values of k, we can have quadratic polynomials all having sum of zeroes as 14 and product of zeroes as -1.

(ii) Sum of zeroes = α + β = √2 = −ba; product of zeroes = αβ = 13 = ca
Quadratic polynomial is ax2 + bx + c
Let a = k,b = -√2k and c = k3
Putting these values we get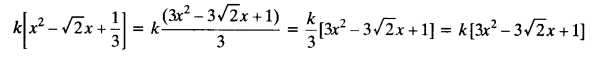

For all different real values of k, we can have different quadratic polynomials of the form 3×2 – 3√2x +1 having sum of zeroes = √2 and product of zeroes = 13

(iii) Sum of zeroes = α + β = 0 = −ba; product of zeroes = αβ = √5 = ca
Let quadratic polynomial is ax2 + bx + c
Let a = k,b = 0, c = √5 k
Putting these values, we get
k[x2 – 0x + √5 ] = k(x2 + √5).
For different real values of k, we can have different quadratic polynomials of the form
x2 + √5, having sum of zeroes = 0 and product of zeroes = √5

(iv) Sum of zeroes = α + β = 1= −ba; product of zeroes = αβ = 1 = ca
Let quadratic polynomial is ax2 + bx + c.
Let a=k, c = k, b = -k
Putting these values, we get k[x2 -x +1]
Quadratic polynomial is of the form x2 -x + 1 for different values of k.

(v) Sum of zeroes = α + β = −14= −ba; product of zeroes = αβ = 14 = ca
Let quadratic polynomial is ax2 + bx + c
Let a=k, b= k4, c= k4
Putting these values, we get k

Quadratic polynomial is of the form 4x2 +x + 1 for different values of k.

(vi) Sum of zeroes = α + β = 4 = −ba; product of zeroes = αβ = 1 = ca
Let quadratic polynomial is ax2 + bx + c
Let a = k,b = -4k and c = k
Putting these values, we get
k[x2 – 4x + 1]
Quadratic polynomial is of the form x2 – 4x + 1 for different values of k.