Vector Algebra Class 12 Maths Important Questions Chapter 10

 

Vector Algebra Class 12 Important Questions with Solutions Previous Year Questions

Algebra of Vectors

Question 1.
Find the position vector of a point which divides the join of points with position vectors a⃗ −2b⃗  and 22a⃗ +b⃗  externally in the ratio 2:1. (Delhi 2016)
Answer:
Let given position vectors are OA−→−=a⃗ −2b⃗  and OB−→−=2a⃗ +b⃗ .

Let OA−→− be the position vector of a point C which divides the join of points, with position vectors
OA−→− and OB−→−, externally in the ratio 2:1.
∴ OA−→− = 2OB−→−−1OA−→−2−1=2(2a⃗ +b⃗ )−1(a⃗ −2b⃗ )1 [by external section formula]
= 4a⃗  + 2b⃗  – a⃗  + 2b⃗  = 3a⃗  + 4b⃗ 

Question 2.
If a⃗  = 4î – ĵ + k̂ and b⃗  = 2î – 2ĵ + k̂, then find a unit vector parallel to the vector a⃗ +b⃗ . (All India 2016)
Answer:
Given vectors are
a⃗  = 4î – ĵ + k̂, b⃗  = 2î – 2ĵ + k̂.
Now, a⃗ +b⃗  == (4î – ĵ + k̂) + (2î – 2ĵ + k̂)
= 6î – 3ĵ + 2k̂

and |a⃗ +b⃗ |=(6)2+(−3)2+(2)2−−−−−−−−−−−−−−−√
= 36+9+4−−−−−−−−√=49−−√ = 7units

∴ The unit vector parallelto the vector a⃗ +b⃗  is
a⃗ +b⃗ |a⃗ +b⃗ |=6i^−3j^+2k^7

Question 3.
The two vectors ĵ + k̂ and 3î – ĵ + 4k̂ represent the two sides AB−→− and AC−→− respectively of triangle ABC. Find the length of the median through A. (Delhi 2016; Foreign 2015)
Answer:
Given, AB−→− = ĵ + k̂ and AC−→− = 3î – ĵ + 4k̂

Alternate Method:
Given 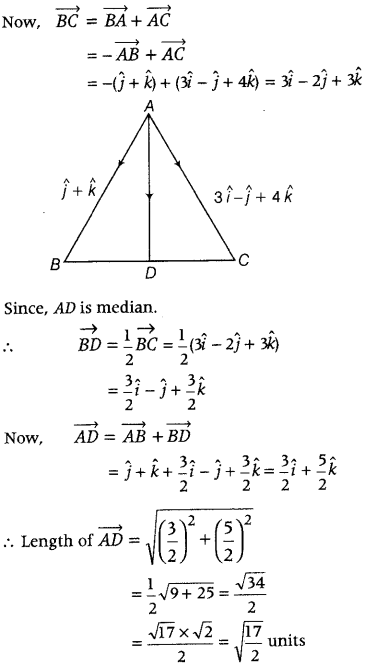AB−→− = ĵ + k̂ and AC−→− = 3î – ĵ + 4k̂

Question 4.
Write the direction ratios of the vector 3a⃗  + 2b⃗ , where a⃗  = î + ĵ – 2k̂ and b⃗  = 2î – 4ĵ + 5k̂ (All India 2015C)
Answer:
Clearly, 3a⃗  + 2b⃗  = 3 (î + ĵ – 2k̂) + 2 (2î – 4ĵ + 5k̂)
= (3î + 3ĵ – 6k̂) + (4î – 8ĵ + 10k̂)
= 7î – 5ĵ + 4k̂
Hence, direction ratios of vectors 3a⃗  + 2b⃗  are 7, – 5 and 4.

Question 5.
Find the unit vector in the direction of the sum of the vectors 2î + 3ĵ – k̂ and 4î – 3ĵ + 2k̂. (Foreign 2015)
Answer:
Let a⃗  = 2î + 3ĵ – k̂ and b⃗  = 4î – 3ĵ + 2k̂
Now, sum of two vectors,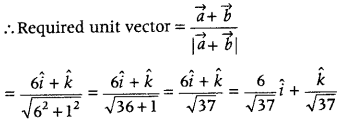
a⃗ +b⃗  = (2î + 3ĵ – k̂) + (4î – 3ĵ + 2k̂) = 6î + k̂

Question 6.
Find a vector in the direction of vector 2î – 3ĵ + 6k̂ which has magnitude 21 units. (Foreign 2014)
Answer:
To find a vector in the direction of given vector, first of all we find unit vector in the direction of given vector and then multiply it with given magnitude.

Let a⃗  = 2î – 3ĵ + 6k̂
Then, |a⃗ | = (2)2+(−3)2+(6)2−−−−−−−−−−−−−−−√
= 4+9+36−−−−−−−−√=49−−√ = 7 units

The unit vector in the direction of the given vector 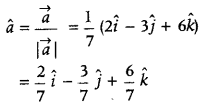a⃗  is

Now, the vector of magnitude equal to 21 units
and in the direction of a is given by
21â = 21(27i^−37j^+67k^) = 6î – 9ĵ + 18k̂

Question 7.
Find a vector a of magnitude 5√2, making an angle of π4 with X-axis, π2 with Y-axis and an acute angle 0 with Z-axis. (All India 2014)
Answer:
Here, we have l = cos π4, m = cos π2 and n = cosθ
⇒ l = 12√, m = 0 and n = cosθ

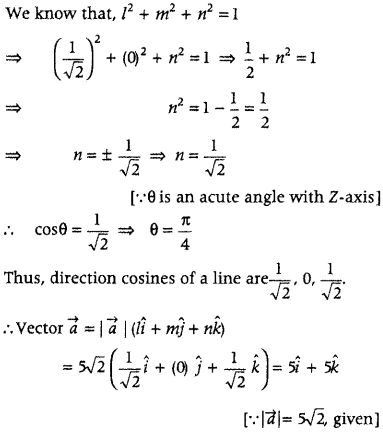
Question 8.
Write a unit vector in the direction of the sum of the vectors a⃗  = 2î + 2ĵ – 5k̂ and b⃗  = -2î + ĵ – 7k̂. (Delhi2014C)
Answer:
113(4î + 3ĵ – 12k̂)

Question 9.
Find the value of p for which the vectors 3î + 2ĵ + 9k̂ and î – 2pĵ + 3k̂ are parallel. (All India 2014)
Answer:
Given, 3î + 2ĵ + 9k̂ and î – 2pĵ + 3k̂ are two parallel vectors, so their direction ratios will be proportional.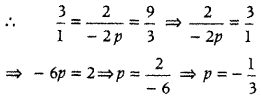

Question 10.
Write the value of cosine of the angle which the vector a⃗  = î + ĵ + k̂ makes with Y-axis. (Delhi 2014C)
Answer:
Given, a⃗  = î + ĵ + k̂
Now, unit vector in the direction of 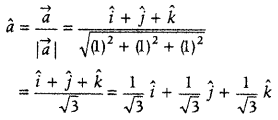a⃗  is

∴ Cosine of the angle which given vector makes with Z-axis is 13√

Question 11.
Find the angle between X-axis and the vector î + ĵ + k̂. (All India 2014C)
Answer:
Let a⃗  = î + ĵ + k̂
Now, unit vector in the direction of a⃗  is

So, angle between X-axis and the vector
î + ĵ + k̂ is cos α = 13√ ⇒ α = cos-1 (13√)
[∵ â = lî + mĵ + nk̂ and cos α = l ⇒ α = cos-1l]

Question 12.
Write a vector in the direction of the vector î – 2ĵ + 2k̂ that has magnitude 9 units. (Delhi 2014C)
Answer:
3î – 6ĵ + 6k̂

Question 13.
Write a unit vector in the direction of vector PQ→, where P and Q are the points (1, 3, 0) and (4, 5, 6), respectively. (Foreign 2014)
Answer:
First, find the vector PQ−→− by using the formula (x2 – x1)î + (y2 – y1)ĵ + (z2 – z1)k̂, then required unit vector is given by PQ−→−|PQ−→−|

Given points are P (1, 3, 0) and Q (4, 5, 6).
Here, x1 = 1, y1 = 3, z1 = 0
and x2 = 4, y2 = 5, z2 = 6
So, vector PQ = (x2 – x1)k̂ + (y2 – y1)ĵ + (z2 – z1)k̂
= (4 – 1)î + (5 – 3)ĵ + (6 – 0)k̂
= 3î + 2ĵ + 6k̂
∴ Magnitude of given vector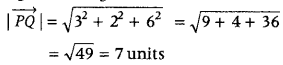

Hence, the unit vector in the direction of 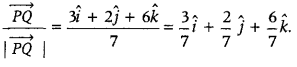PQ→ is

Question 14.
If a unit vector a⃗  makes angle π3 with î, π4 with ĵ and an acute angle θ with k̂, then find the value of θ. (Delhi 2013)
Answer:
Here, we have
l = cos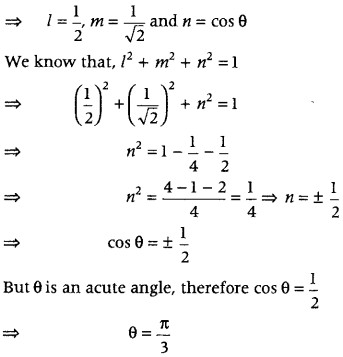π3, m = cos π4 and n = cos θ

Question 15.
Write a unit vector in the direction of the sum of vectors a⃗  = 2î – ĵ + 2k̂ and b⃗  = – î + ĵ + 3k̂. (Delhi 2013)
Answer:
126√i^+526√k^

Question 16.
If a⃗  = xî +2ĵ – zk̂ and b⃗  = 3î – yĵ + k̂ are two equal vectors, then write the value of x + y + z. (Delhi 2013)
Answer:
Two vectors are equal, if coefficients of their components are equal.
Given, a⃗ =b⃗  ⇒ xî + 2ĵ – zk̂ = î – yĵ + k̂

On comparing the coefficient of components, we get
x = 3, y = -2, z = -1
Now, x + y + z = 3 – 2 – 1 = 0

Question 17.
P and Q are two points with position vectors 3a⃗  – 2b⃗  and a⃗  + b⃗ , respectively. Write the position vector of a point R which divides the line segment PQ in the ratio 2 : 1 externally. (All India 2013)
Answer:
−a⃗ +4b⃗ 

Question 18.
L and M are two points with position vectors 2a⃗  – b⃗  and a⃗  + 2b⃗ , respectively. Write the position vector of a point N which divides the line segment LM in the ratio 2 : 1 externally. (All India 2013)
Answer:
5b⃗ 

Question 19.
A and B are two points with position vectors 2a⃗  – 3b⃗  and 6b⃗  – a⃗ , respectively. Write the position vector of a point P which divides the line segment AB internally in the ratio 1:2. (All India 2013)
Answer:
Given, A and B are two points with position vectors 2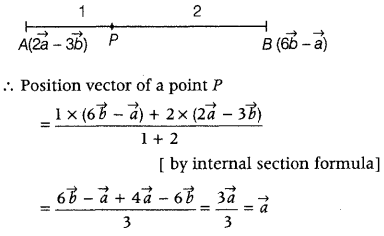a⃗  – 3b⃗  and 6b⃗  – a⃗ , respectively. Also, point P divides the line segment AB in the ratio 1 : 2 internally.

Question 20.
Find the sum of the vectors a⃗  = î – 2ĵ + k̂ b⃗  = – 2î + 4ĵ + 5k̂ and c⃗  = î – 6ĵ – 7k̂. (Delhi 2012)
Answer:
Given vectors are a⃗  = î – 2ĵ + k̂ b⃗  = – 2î + 4ĵ + 5k̂ and c⃗  = î – 6ĵ – 7k̂.
Sum of the vectors a⃗ , b⃗  and c⃗  is
a⃗ +b⃗ +c⃗  = (î – 2ĵ + k̂) + (- 2î + 4ĵ + 5k̂) + (î – 6ĵ – 7 k̂)
= – 4ĵ – k̂

Question 21.
Find the sum of the following vectors. a⃗  = î – 3k̂, b⃗  = 2ĵ – k̂, c⃗  = 2î – 3ĵ + 2k̂. (Delhi 2012)
Answer:
3î – ĵ – 2k̂

Question 22.
Find the sum of the following vectors. a⃗  = î – 2ĵ, b⃗  = 2î – 3 ĵ, c⃗  = 2î + 3k̂. (Deihi 2012)
Answer:
5î – 5ĵ + 3k̂

Question 23.
Find the scalar components of AB→ with initial point A (2,1) and terminal point B(- 5, 7). (All India 2012)
Answer:
Given initial point is A (2,1) and terminal point is B (- 5, 7), then scalar component of AB−→− are
x2 – x1 = – 5 – 2 = – 7and y2 – y1 = 7 – 1 = 6.

Question 24.
For what values of a⃗ , the vectors 2î – 3ĵ + 4k̂ and aî + 6ĵ – 8k̂ are collinear? (Delhi 2011)
Answer:
If a⃗  and b⃗  are collinear, then use the condition a⃗  = λb⃗ , where λ is some scalar.

Let given vectors are a⃗  = 2î – 3ĵ + 4k̂ and a⃗  = aî + 6ĵ – 8k̂
We know that, vectors a⃗  and b⃗  are said to be collinear, if
a⃗  = k. b⃗ , where k is a scalar.
∴ 2î – 3ĵ + 4k̂ = k(aî + 6ĵ – 8k̂)

On comparing the coefficients of î and ĵ, we get
2 = ka and -3 = 6k ⇒ k = –12
∴ 2 = –12a ⇒ a = -4

Question 25.
Write the direction cosines of vector -2î + ĵ – 5k̂. (Delhi 2011)
Answer:
Direction cosines of the vector aî + bĵ + ck̂ are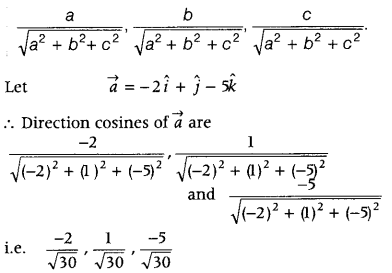

Question 26.
Write the position vector of mid-point of the vector joining points P(2, 3, 4) and Q (4, 1, – 2). (Foreign 2011)
Answer:
Mid-point of the position vectors
a⃗  = a1î + a2ĵ + a3k̂ and
b⃗  = b1î + b2ĵ + b3k̂ is a⃗ +b⃗ 2 or (a1+b1)i^+(a2+b2)j^+(a3+b3)k^2

Given points are P(2, 3, 4) and Q(4,1,-2) whose position vectors are OP−→− = 2 î + 5ĵ + 4k̂ and OQ−→− =4î + ĵ – 2k̂.
Now, position vector of mid-point of vector joining points P(2, 3, 4) and Q(4, 1, – 2) is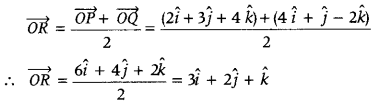

Question 27.
Write a unit vector in the direction of vector a⃗  = 2î + ĵ + 2k̂. (All India 2011; Delhi 2009)
Answer:
We know that, unit vector in the direction of â is â = a⃗ |a⃗ |

Required unit vector in the direction of vector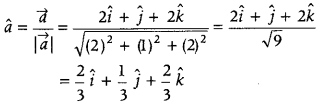
a⃗  = 2î + ĵ + 2k̂

Question 28.
Find the magnitude of the vector a⃗  = 3î – 2ĵ + 6k̂. (All India 2011C: Delhi 2008)
Answer:
Magnitude of a vector r = xî + yĵ + zk̂ is |r⃗ | = x2+y2+z2−−−−−−−−−−√

Given vector is a = 3i – 2/ + 6fc.
∴ Magnitude of a⃗  = |a⃗ |=(3)2+(−2)2+(6)2−−−−−−−−−−−−−−−√
= 9+4+36−−−−−−−−√=49−−√ = 7 units

Question 29.
Find a unit vector in the direction of vector a⃗  = 2î + 3ĵ + 6k̂. (All India 2011C)
Answer:
27i^+37j^+67k^

Question 30.
If A, B and C are the vertices of a ΔABC, then what is the value of AB−→−+BC−→−+CA−→− ? (Delhi 2011C)
Answer:
Let ΔABC be the given triangle.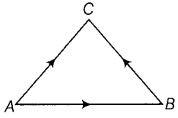

Now, by triangle law of vector addition,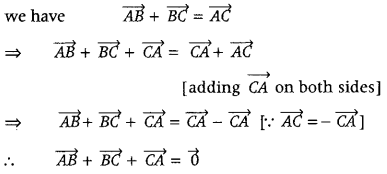

Question 31.
Find a unit vector in the direction of a⃗  = 2î – 3ĵ + 6k̂. (Delhi 2011c)
Answer:
27i^−37j^+67k^

Question 32.
Find a vector in the direction of a⃗  = 2î – ĵ + 2k̂, which has magnitude 6 units. (Delhi 2010C)
Answer:
4î – 2ĵ + 4k̂

Question 33.
Find the position vector of mid-point of the line segment AB, where A is point (3, 4, -2) and Bis point (1, 2, 4). (Delhi 2010)
Answer:
2î + 3ĵ + k̂

Question 34.
Write a vector of magnitude 9 units in the direction of vector -2î + ĵ + 2k̂. (All India 2010)
Answer:
-6î + 3ĵ + 6k̂

Question 35.
Write a vector of magnitude 15 units in the direction of vector î – 2ĵ + 2k̂. (Delhi 2010)
Answer:
5î – 10ĵ + 10k̂

Question 36.
What is the cosine of angle which the vector √2î + ĵ + k̂ makes with Y-axis? (Delhi 2010)
Answer:
12

Question 37.
Find a vector of magnitude 5 units and parallel to the resultant of a⃗  = 2î + 3ĵ – k̂ and b⃗  = î – 2ĵ + k̂. (Delhi 2011)
Answer:
First, find resultant of the vectors a and o, which is a⃗  + b⃗ . Then, find a unit vector in the direction of a⃗  + b⃗ . After this, the unit vector is multiplying by 5.

Given, a⃗  = 2î + 3ĵ – k̂ and b⃗  = î – 2ĵ + k̂.
Now, resultant of above vectors = a⃗  + b⃗ 
= (2î + 3ĵ – k̂) + (î – 2ĵ + k̂) = 3î + ĵ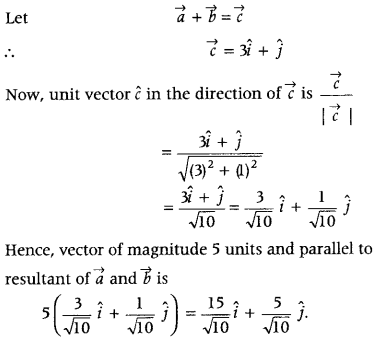

Question 38.
Let a⃗  = î + ĵ + k̂, b⃗  = 4î – 2ĵ + 8k̂ and c⃗  = î – 2ĵ + k̂. Find a vector of magnitude 6 units, which is parallel to the vector 2a⃗  – b⃗  + 8 c⃗ . (All India 2010)
Answer:
First, find the vector 2 a – b + 3c, then find a unit vector in the direction of 2a-b + 3c.
After this, the unit vector is multiplying by 6.
Given, a⃗  = î + ĵ + k̂, b⃗  = 4î – 2ĵ + 8k̂ and c⃗  = î – 2ĵ + k̂
∴ 2a⃗ −b⃗ +3c⃗ 
= 2 (î + ĵ + k̂) – (4î – 2ĵ + 3k̂) + 3 (î – 2ĵ + k̂)
= 2î + 2ĵ + 2k̂ – 4î + 2ĵ – 3k̂ + 3î – 6ĵ + 3k̂
⇒ 2a⃗ −b⃗ +3c⃗  = î – 2ĵ + 2k̂

Now, a unit vector in the direction of vector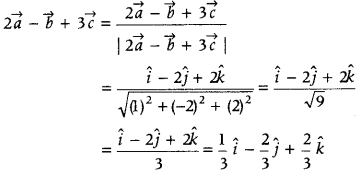

Hence, vector of magnitude 6 units parallel to the Vector 2a⃗ −b⃗ +3c⃗  = 6(13i^−23j^+23k^)
= 2î – 4ĵ + 4k̂

Question 39.
Find the position vector of a point R, which divides the line joining two points P and Q whose position vectors are 2a⃗  + b⃗  and a⃗  – 8b⃗  respectively, externally in the ratio 1 : 2. Also, show that P is the mid-point of line segment RO. (Delhi 2010)
Answer:
Given, OP−→− = Position vector of P = 2a⃗  + b⃗ 
and OQ−→− = Position vector of Q = a⃗  – 3b⃗ 

Let OR be the position vector of point R, which divides PQ in the ratio 1 : 2 externally

Now, we have to show that P is the mid-point of RQ,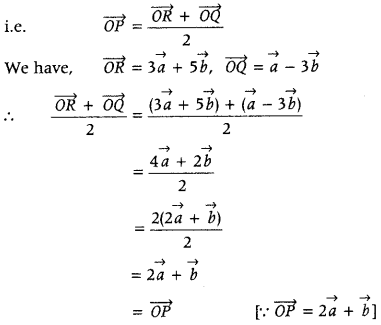

Hence, P is the mid-point of line segment RQ.

Product of Two Vectors and Scalar Triple Product

Question 1.
Find the magnitude of each of the two vectors a⃗  and b⃗ , having the same magnitude such that the angle between them is 60° and their scalar product is 92. (CBSE 2018)
Answer:

Question 2.
Find the value of [î, k̂, ĵ], (CBSE 2018C)
Answer:
[î, k̂, ĵ] = î ∙ (k̂× ĵ)
= -[[î, k̂, ĵ] = –∣∣∣∣100010001∣∣∣∣ = – 1

Question 3.
Find λ and μ, if (î + 3ĵ + 9k̂) × (3î – λĵ + μk̂) = 0. (All India 2016)
Answer:
Given, (î + 3ĵ + 9k̂) × (3î – λĵ + μk̂)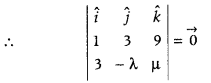

= î(3μ + 9λ) ĵ k̂
On comparing the coefficients of î, ĵ and k̂ , we get
3μ + 9λ = 0, – μ + 27 = 0 and – λ – 9 = 0
⇒ μ = 27 and – λ = 9
⇒ μ = 27 and λ = – 9
Also, the values of μ and λ satisfy the equation
3μ + 9λ = 0.
Hence, μ = 27 and λ = – 9.

Question 4.
Write the number of vectors of unit length perpendicular to both the vectors a⃗  = 2î + ĵ + 2k̂ and b⃗  = ĵ + k̂. (All India 2016)
Answer:
We know that, unit vectors perpendicular to a⃗ 
and b⃗  are ±(a⃗ ×b⃗ |a⃗ ×b⃗ |)
So, there arc two unit vectors perpendicular to the given vectors.

Question 5.
If a⃗ ,b⃗ ,c⃗  are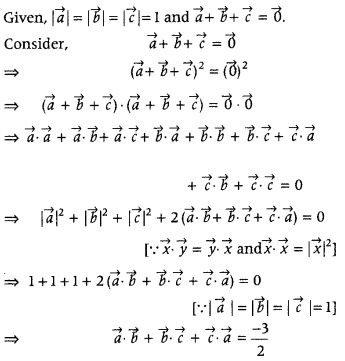 unit vectors such that a⃗ +b⃗ +c⃗ =0→ = 0, then write the value of a⃗ ⋅b⃗ +b⃗ ⋅c⃗ +c⃗ ⋅a⃗ . (Foreign 2016)
Answer:

Question 6.
If |a⃗ ×b⃗ |2+|a⃗ ⋅b⃗ |2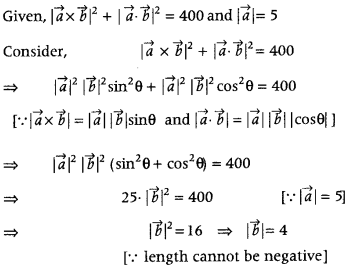 = 400 and |a⃗ | = 5, then write the value of |b⃗ |. (Foreign 2016)
Answer:

Question 7.
Find λ, if the vectors a⃗  = î + 3ĵ + k̂, b⃗  = 2 î – ĵ – k̂ and c⃗  = λĵ + 3 k̂ are coplanar. (Delhi 2015)
Answer:

⇒ 1(- 3 + λ) – 3(6) + 1(2λ) = 0
[expanding along R1]
⇒ – 3 + λ – 18 + 2λ = 0
⇒ 3λ = 21
∴ λ = 7

Question 8.
If a⃗  = 7î + ĵ – 4k̂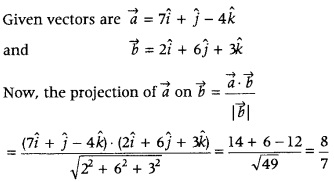 and b⃗  = 2î + 6ĵ + 3k̂, then find the projection of a⃗  on b⃗ . (Delhi 2015)
Answer:

Question 9.
If â, b̂ and ĉ are mutually perpendicular unit vectors, then find the value of |2â + b̂ + ĉ |. (All India 2015)
Answer:
Given â, b̂ and ĉ are mutually perpendicular unit vectors, i.e.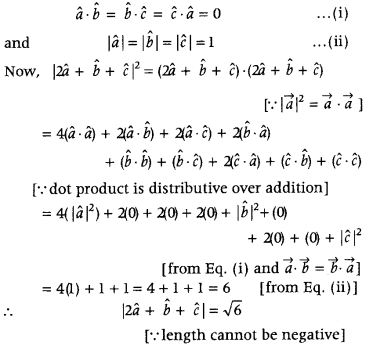

Question 10.
Write a unit vector perpendicular to both the vectors a⃗  = î + ĵ + k̂ and b⃗  = î + ĵ. (All India 2015)
Answer:
First, determine perpendicular vectors of a⃗  and b⃗ , i.e., a⃗ ×b⃗ . Further , determine perpendicular unit vector by using formula a⃗ ×b⃗ |a⃗ ×b⃗ |.

Given vector are a⃗  = î + ĵ + k̂ and b⃗  = î + ĵ
As we know the, vectors a⃗ ×b⃗  is perpendicular to both the vectors, so let us first evaluate a⃗ ×b⃗ .
Then, a⃗ ×b⃗  = ∣∣∣∣∣i^11j^11k^10∣∣∣∣∣
= î(0 -1) – ĵ(0 – 1) + k̂(1 – 1)
= – î + ĵ
Then , the unit vector perpendicular to both 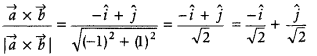a⃗  and b⃗  is given by

Question 11.
Find the area of a parallelogram whose adjacent sides are represented by the vectors 2 î – 3 k̂ and 4 ĵ + 2 k̂. (Foreign 2015)
Answer:
Let adjacent sides of a parallelogram bc
a⃗  = 2 î – 3 k̂ and b⃗  = 4 ĵ + 2 k̂.

Question 12.
If a⃗  and b⃗  a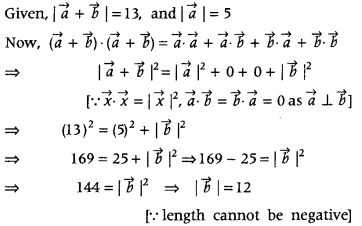re perpendicular vectors, |a⃗  + b⃗ | = 13 and |a⃗ | = 5, then find the value of |b⃗ |. (All India 2014)
Answer:

Question 13.
If a⃗  and b⃗  a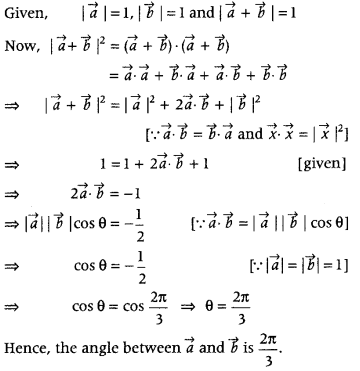re two unit vectors such that a⃗  + b⃗  is also a unit vector, then find the angle between a⃗  and b⃗ . (Delhi 2014)
Answer:

Question 14.
Find the projection of the vector î + 3ĵ + 7k̂ on the vector 2î – 3 ĵ + 6k̂. (Delhi 2014)
Answer:
let a⃗  = î + 3ĵ + 7k̂ and a⃗  = 2î – 3 ĵ + 6k̂

Question 15.
Write the projection of vector î + ĵ + k̂ along the vector ĵ. (Foreign 2014)
Answer:
1

Question 16.
Write the value of the following. î × (ĵ + k̂) + ĵ × (k̂ + î) + k̂ × (î + ĵ). (Foreign 2014)
Answer:
we have, î × (ĵ + k̂) + ĵ × (k̂ + î) + k̂ × (î + ĵ)
= î × ĵ + î × k̂ × ĵ × k̂ + ĵ × î + k̂ × î + k̂ × ĵ
[∵ cross product is distributive over addition]
= k̂ – ĵ + î – k̂ + ĵ – î = 0⃗ 
[∵ î × ĵ = k̂, î × k̂ = – ĵ, ĵ × k̂ = î, ĵ × î = – k̂, k̂ × î = ĵ, k̂ × ĵ = – î ]

Question 17.
If vectors a⃗  and b⃗  are such that |a⃗ | = 3, |b⃗ | = 2/3 and a⃗  × b⃗  is a unit vector, then write the angle between a⃗  and b⃗ . (Delhi 2014: All India 2010)
Answer: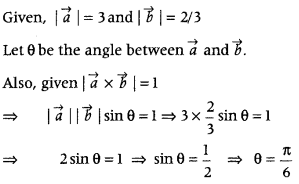

Question 18.
Find a⃗ ⋅(b⃗ ×c⃗ ), if a⃗  = 2î + ĵ + 3k̂, b⃗  = -î + 2ĵ + k̂, and c⃗  = 3î + ĵ + 2k̂. (All India 2014)
Answer:
Given, 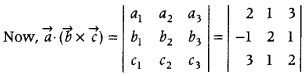a⃗  = 2î + ĵ + 3k̂, b⃗  = -î + 2ĵ + k̂, and c⃗  = 3î + ĵ + 2k̂.

= 2(4 – 1) – 1 (- 2 – 3) + 3( – 1 – 6)
= 2 × 3 – 1 × (-5) + 3 × (- 7)
= 6 + 5 – 21 = 11 – 21 = – 10

Question 19.
If a⃗  and b⃗  a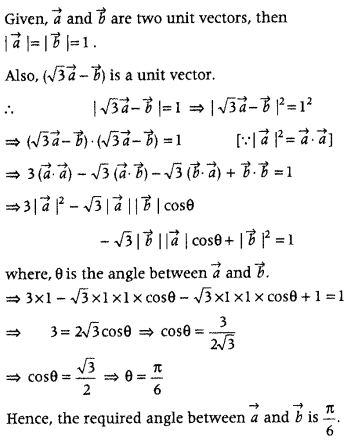re unit vectors, then find the angle between a⃗  and b⃗ , given that (√3a⃗  – b⃗ ) is a unit vector. (Delhi 2014C)
Answer:

Question 20.
If |a⃗ | = 8, |b⃗ | = 3 and||a⃗ ×b⃗ || = 12, find the angle between a⃗  and b⃗ . (All India 2014C)
Answer:
let θ be the angle between latex]\vec{a}[/latex] and b⃗ .

Question 21.
Write the projection of the vector a⃗  = 2 î – ĵ + k̂ on the vector b⃗  = î + 2ĵ + 2k̂. (Delhi 2014 C)
Answer:
23

Question 22.
Write the value of λ, so that the vectors a = 2î + λĵ + k̂ and b = î – 2ĵ + 3k̂ are perpendicular to each other. (Delhi 2013C, 2008)
Answer:
Given vectors are a⃗  = 2î + λĵ + k̂
and b⃗  = î – 2ĵ + 3k̂
Since, vectors are perpendicular.
∴ a⃗ ⋅b⃗  = 0
⇒ (2î + λĵ + k̂) ∙ (î – 2ĵ + 3k̂)
⇒ 2 – 2λ + 3 = 0
∴ λ = 5/2

Question 23.
Write the projection of (b⃗  + c⃗ ) on a⃗ , where a⃗  = 2î – 2ĵ + k̂, b⃗  = î + 2ĵ – 2k̂ and c⃗  = 2î – ĵ + 4k̂. (All India 2013 C)
Answer: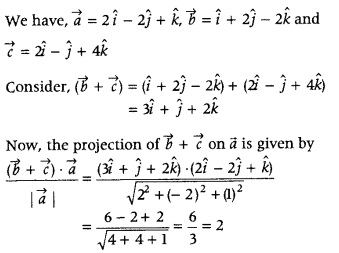

Question 24.
Write the projection of the vector 7î + ĵ – 4k̂ on the vector 2î + 6 ĵ + 3k̂. (Delhi 2013C)
Answer:
87

Question 25.
If a⃗  and b⃗  a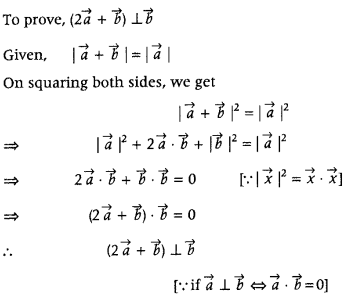re two vectors such that |a⃗  + b⃗ | = |a⃗ |, then prove that vector 2a⃗  + b⃗  is perpendicular to vector b. (Delhi 2013)
Answer:

Question 26.
Find |x⃗ |, if for â unit vector a, (x⃗ −a⃗ )⋅(x⃗ +a⃗ ) = 15. (All India 2013)
Answer:
Given, 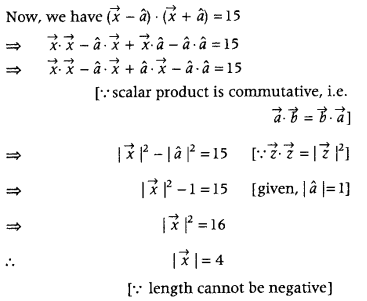a⃗  is a unit vector. Then, |a⃗ | = 1.

Question 27.
Find λ, when projection of a⃗  = λî + ĵ + 4k̂ on b⃗  = 2 î + 6 ĵ + 3k̂ is 4 units. (Delhi 2012)
Answer:
Given, 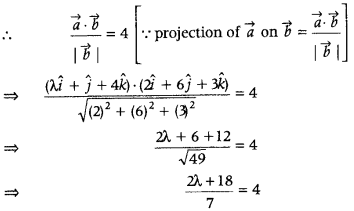a⃗  = λî + ĵ + 4k̂ on b⃗  = 2 î + 6 ĵ + 3k̂ and projection of a⃗  and b⃗  = 4.

⇒ 2λ + 18 = 28
⇒ 2λ = 10
∴ λ = 5

Question 28.
Write the value of (k̂ × ĵ) . î + ĵ . k̂. (All India 2012)
Answer:
Use the results k̂ × ĵ = – î
ĵ ∙ k̂ and î ∙ î = 1 and simplify it.

Given, (k̂ × î) ∙ î + ĵ ∙ k̂ = (- î) ∙ î + ĵ ∙ k̂
= – (î ∙ î) + 0 = – 1 [∵ (î ∙ î) = 1]

Question 29.
If a⃗ ⋅a⃗  = 0 and a⃗ ⋅b⃗  = 0, then what can be concluded about the vector b⃗ ? (Foreign 2011)
Answer: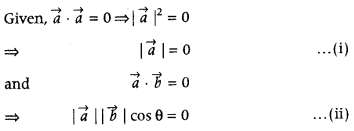

From Eqs. (i) and (ii). it may be concluded that b⃗  is either zero or non-zero perpendicular vector.

Question 30.
Write the projection of vector î – ĵ on the vector î + ĵ. (All India 2011)
Answer:
0

Question 31.
Write the angle between vectors a⃗  and b⃗  with magnitudes √3 and 2 respectively, having a⃗ . b⃗  = √6. (All India 2011)
Answer:
let θ be the angle between a⃗  and b⃗ , then use the following formula
cos θ = a⃗ ⋅b⃗ |a⃗ ||b⃗ |.

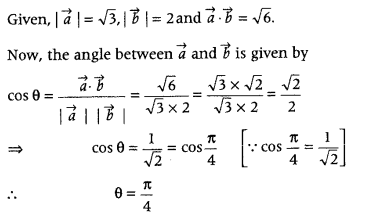
Question 32.
For what value of λ are the vectors î + 2λĵ + k̂ and 2î + ĵ – 3k̂ perpendicular? (All India 2011C)
Answer:
12

Question 33.
If |a⃗ | = √3, |b⃗ | = 2 and angle between a⃗  and b⃗  is 60°, then find a⃗ .b⃗ . (Delhi 2011C)
Answer:

Question 34.
Find the value of λ, if the vectors 2î + λĵ + 3k and 3î + 2ĵ – 4k̂ are perpendicular to each other. (All India 2010c)
Answer:
3

Question 35.
If |a⃗ | = 2, |b⃗ | = 3 an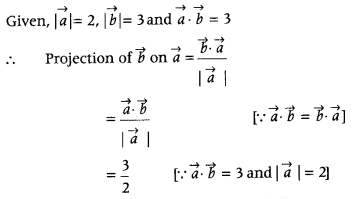d a⃗ .b⃗  = 3, then find the projection of b⃗  on a⃗ . (All India 2010C)
Answer:

Question 36.
If a⃗  and b⃗  are two vectors, such that |a⃗ ⋅b⃗ |=|a⃗ ×b⃗ |, then find the angle between a⃗  and b⃗ . (All India 2010)
Answer:
Use the following formulae:
a⃗ ⋅b⃗  = |a⃗ ||b⃗ | cos θ
and |a⃗ ×b⃗ | = |a⃗ ||b⃗ | sin θ
where, θ is the angle between 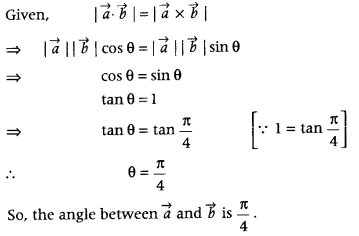a⃗  and b⃗ .

Question 37.
Find λ, if (2î + 6ĵ + 14k̂) × (î – λĵ + Ik̂) = 0. (All India 2010)
Answer:
– 3

Question 38.
If the sum of two unit vectors a and b is a unit vector, show that the magnitude of their difference is √3. (Delhi 2019, 2012c)
Answer:
let c⃗  = a⃗  + b⃗ . Then, according to given condition c⃗  is a unit vector, i.e. |c⃗ | = 1.

[taking positive square root, as magnitude cannot be negative]

Question 39.
If a⃗  = 2î + 5ĵ + k̂, b⃗  = î – 2 ĵ + k̂ and c⃗  = – 3î + ĵ + 2k̂, find [a⃗ b⃗ c⃗ ]. (Delhi 2019)
Answer: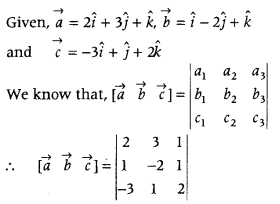

= 2(- 4 – 1) – 3(2 + 3) + 1(1 – 6)
= – 10 – 15 – 5 = – 30

Question 40.
If |a⃗ | = 2, |b⃗ | = 7 and a⃗ ×b⃗  = 3î + 2ĵ + 6k̂, find the angle between a⃗  and b⃗ . (All India 2019)
Answer:
let θ be the angle between 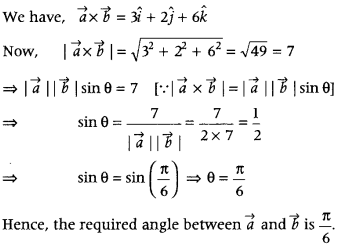a⃗  and b⃗ .

Question 41.
Find the volume of cuboid whose edges are given by -5î + 7ĵ + 5k̂, -5î + 7ĵ – 5k̂ and 7î – 5 ĵ – 5k̂. (All India 2019)
Answer:

= |- 3 (- 21 – 15) – 7 (15 + 21) + 5(25 – 49)|
= |1108 – 252 – 120|
= 264 cubic units

Question 42.
Show that the points A(-2î + 5ĵ + 5k̂), B(î + 2 ĵ + 5k̂) and C(7î – k̂) are collinear. (All India 2019)
Answer: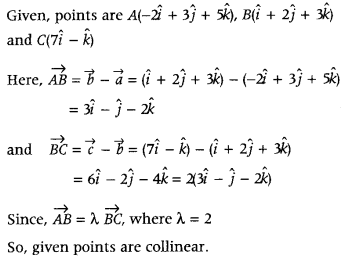

Question 43.
Find |a⃗ ×b⃗ |, if a⃗  = 2î + ĵ + 5k̂ and b⃗  = 3î + 5ĵ – 2k̂. (All India 2019)
Answer:
We have, a⃗  = 2î + ĵ + 3k̂ and b⃗  = 3î + 5ĵ – 2k̂
∴ a⃗ ×b⃗  = ∣∣∣∣∣i^23j^15k^3−2∣∣∣∣∣
= î ( – 2 – 15) – ĵ (- 4 – 9) + k̂(10 – 3)
= – 17î + 13ĵ + 7k̂

Question 44.
If θ is the angle between two vectors î – 2 ĵ + 3k̂ and 3î – 2 ĵ + k̂, find sin θ. (CBSE 2018)
Answer:
let a⃗  = î – 2 ĵ + 3k̂ and b⃗  3î – 2 ĵ + k̂

Question 45.
If a⃗ +b⃗ +c⃗  = 0 and |a⃗ | = 5, |b⃗ | = 6 and |c⃗ | = 9, then find the angle between a⃗  and b⃗ . (CBSE 2018C)
Answer: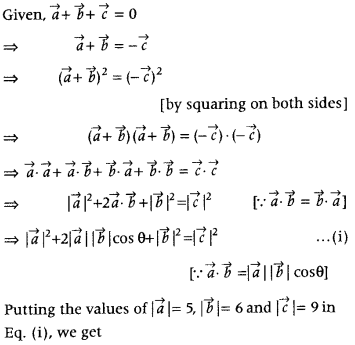

(5)2 + 2 × 5 × 6 × cos θ + (6)2 = (9)2
⇒ 25 + 60 cos θ + 36 = 81
⇒ cos θ = 2060=13
⇒ θ = cos-1(13)

Question 46.
If î + ĵ + k̂, 2î + 5ĵ, 5î + 2ĵ – 5k̂ and î – 6ĵ – k̂ respectively, are the position vectors of points A, B, C and D, then find the angle between the straight lines AB and CD. Find whether AB−→− and C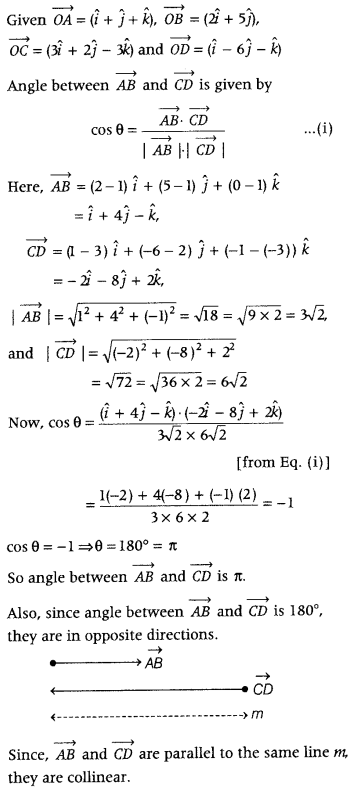D−→− are collinear or not. (Delhi 2019)
Answer:

Question 47.
The scalar product of the vector a⃗  = î + ĵ + k̂ with a unit vector along the sum of the vectors b⃗  = 2î + 4ĵ – 5k̂ and c⃗  = λî + 2ĵ + 5k̂ is equal to 1. Find the value of λ and hence find the unit vector along b⃗  + c⃗ . (All India 2019)
Answer: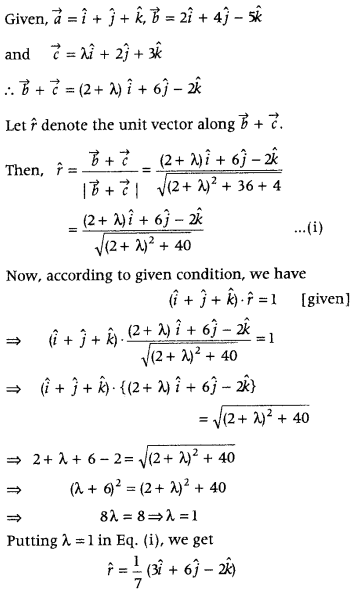

Question 48.
Let a⃗  = 4 î + 5ĵ – k̂, b⃗  = î – 4ĵ + 5k̂ and c⃗  = 3î + ĵ – k̂. Find a vector which is perpendicular to both c⃗  and b⃗  and d⃗ ⋅a⃗  = 21. (CBSE 2018)
Answer:
We have, a⃗  = 4 î + 5ĵ – k̂, b⃗  = î – 4ĵ + 5k̂ and c⃗  = 3î + ĵ – k̂.
Since, d⃗  is perpendicular to both c⃗  and b⃗ .

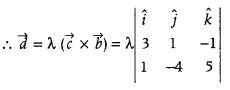
= λ[î(5 – 4) – ĵ(15 + 1) + k̂(- 12 – 1)]
= λ(î – 16ĵ – 13k̂)
Also, it is given that a⃗ ⋅a⃗  = 21
∴ λ(î – 16ĵ – 13k̂) ∙ (4î + 5ĵ – k̂) = 21
⇒ λ(4 – 80 + 13) = 21
⇒ λ(- 63) = 21
⇒ λ = −13
Now from Eq. (j), we get
d⃗  = –−13(î – 16ĵ – 13k̂)

Question 49.
Find x such that the four points A(4, 4, 4), B(5, x, 8), C(5, 4, 1) and D (7, 7, 2) are coplanar. (CBSE 2018C)
Answer:
Given points are A(4, 4, 4), B (5, x, 8), C(5, 4, 1) and D(7, 7, 2), then position vectors of A, B, C and D respectively, are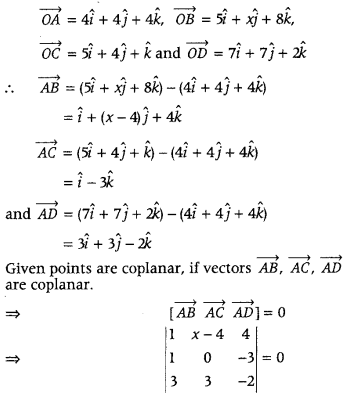

⇒ 1(0 + 9) – (x – 4) (- 2 + 9) + 4(3 – 0) = 0
⇒ 9 – (x – 4) (7) + 12 = 0
⇒ 9 – 7x + 28 + 12 = 0
⇒ 49 – 7x = 0
⇒ 7x = 49
⇒ x = 7

Question 50.
Find the value of x such that the points A(3, 2, 1), B(4, x, 5), C(4, 2,- 2) and D (6, 5, -1) are coplanar. (All India 2017)
Answer:
5

Question 51.
If a⃗ , b⃗  and c⃗  are three mutually perpendicular vectors of the same magnitude, then prove that a⃗ +b⃗ +c⃗  is equally inclined with the vectors a⃗ , b⃗  and c⃗ . (Delhi 2017, 2013C, 2011)
Answer:
If three vectors a⃗ , b⃗  and c⃗  are mutually perpendicular to each other, then a⃗ ⋅b⃗ =b⃗ ⋅c⃗  = c⃗ ⋅a⃗  = 0 and if all three vectors a⃗ , b⃗  and c⃗  are equally inclined with the vector (a⃗ +b⃗ +c⃗ ) that means each vector a⃗ , b⃗  and c⃗  makes equal angle with (a⃗ +b⃗ +c⃗ ) by using formula
cos θ = a⃗ ⋅b⃗ |a⃗ ||b⃗ |.

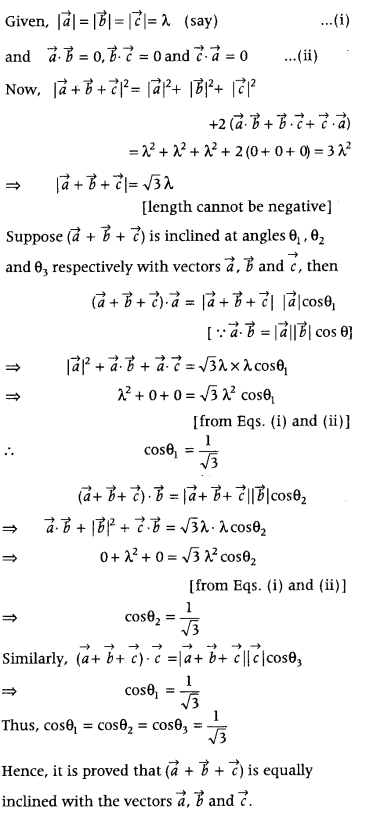
Question 52.
Using vectors, find the area of the ΔABC, whose vertices are A(1, 2, 5), 5(2, -1, 4) and C(4, 5, -1). (Delhi 2017; All India 2013)
Answer:
Let the position vectors of the verices A, B and C of ΔABC be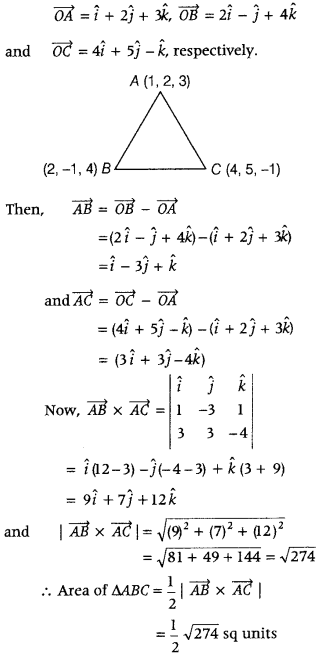

Question 53.
Let a⃗  = î + ĵ + k̂, b⃗  = î + 0 ∙ ĵ + 0 ∙ k̂ and c⃗  = c1î + c2ĵ + c3k̂, then
(a) Let c1 = 1 and c2 = 2, find c3 which makes a⃗ , b⃗  and c⃗  coplanar.
(b) If c2 = – 1 and c3 = 1, show that no value of c1 can make a⃗ , b⃗  and c⃗  coplanar. (Delhi 2017)
Answer:
Given, a⃗  = î + ĵ + k̂, b⃗  = î + 0 ∙ ĵ + 0 ∙ k̂ and c⃗  = c1î + c2ĵ + c3k̂
The given vectors are coplanar iff [a⃗ b⃗ c⃗ ] = 0

(a) If c1 = 1 and c2 = 2,
Then, from Eq.(i), we get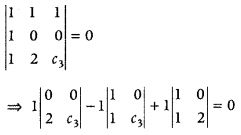
∣∣∣∣11110210c3∣∣∣∣ = 0

⇒ – 1(c3 – 0) + 1(2 – 0) = 0
⇒ – c3 + 2 = 0
⇒ – c3 = – 2
⇒ c3 = 2

(b) If c2 = – 1 and c3 = 1, then from Eq. (i), we get
∣∣∣∣11c110−1101∣∣∣∣ = 0
⇒ 1(0) – 1(1 – 0) + 1(- 1 – 0) = 0
⇒ 0 – 1 – 1 = 0
⇒ – 2 ≠ 0
∴ No value of c1 can make 1’ and coplanar.
Hence proved

Question 54.
Show that the points A, B, C with position vectors 2î – ĵ + k̂, î – 5ĵ – 5k̂ and 5î – 4ĵ – 4k̂ respectively, are the vertices of a right-angled triangle. Hence find the area of the triangle. (All India 2017)
Answer:

Question 55.
Show that the vectors a⃗ , b⃗  and c⃗  are coplanar, if a + b, 6+ c and c+ a are coplanar. (Delhi 2016, Foreign 2014)
Or
Prove that, for any three vectors a⃗ , b⃗  and c⃗ ,[a⃗ +b⃗ b⃗ +c⃗ c⃗ +a⃗ ]=2[a⃗ b⃗ c⃗ ]. (Delhi 2014)
Answer: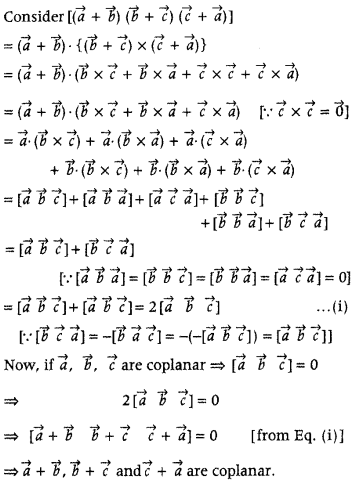

Question 56.
Show that the four points A (4, 5, 1), B(0, -1, -1), C(3, 9, 4) and D (-4, 4, 4) are coplanar. (All India 2016)
Or
Show that the four points A, B, C and D with position vectors 4î + 5ĵ + k̂, – ĵ – k̂, 3î + 9ĵ + 4k̂ and 4(- î + ĵ + k̂), respectively are coplanar. (All India 2014)
Answer:
Let the position vector of points A, B, C and D are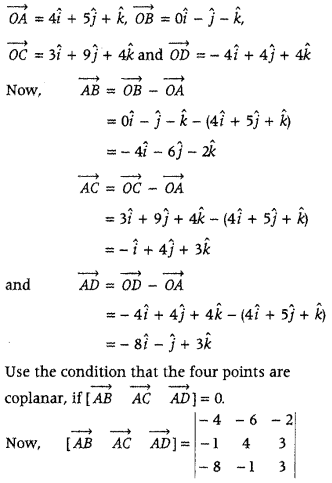

= – 4(12 + 3) + 6 (- 3 + 24) – 2(1 + 32)
= – 60 + 126 – 66 = 0
Hence, the four points A, B, C and D are coplanar.

Question 57.
The two adjacent sides of a parallelogram are 2î – 4ĵ – 5k̂ and 2î + 2 ĵ + 3k̂. Find the two unit vectors parallel to its diagonals. Using the diagonal vectors, find the area of the parallelogram. (All India 2016)
Answer:
Let ABCD be the given parallelogram with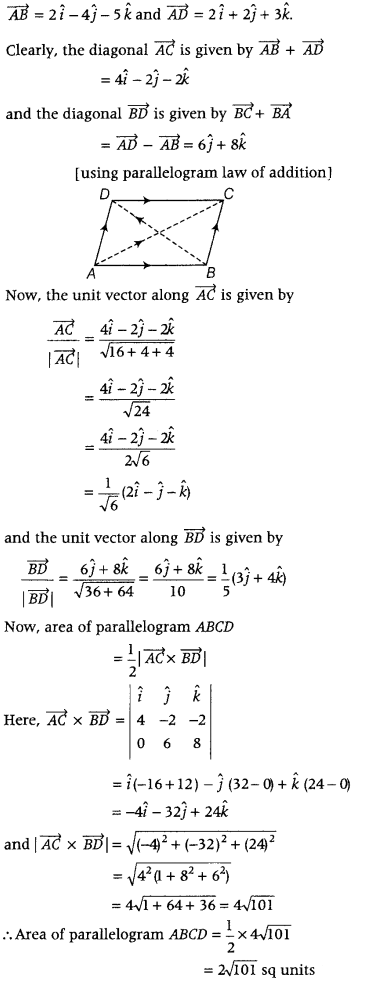

Question 58.
If a⃗ ×b⃗ =c⃗ ×d⃗  and a⃗ ×c⃗ =b⃗ ×d⃗ , then show that a⃗ −d⃗  is parallel to b⃗ −c⃗ , where a⃗ ≠d⃗  and b⃗ ≠c⃗ . (Foreign 2016; Delhi 2009)
Answer:
Use the result, if two vectors are parallel, then their cross-product will be a zero vector.

Question 59.
If r⃗  = xî + yĵ + zk̂, find (r⃗ ×i^)⋅(r⃗ ×j^) + xy. (Delhi 2015)
Answer:

Question 60.
If a⃗  = î + 2ĵ + k̂, b⃗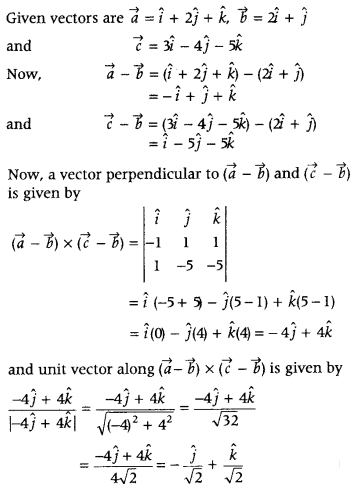  = 2î + ĵ and c⃗  = 3î – 4 ĵ – 5k̂, then find a unit vector perpendicular to both of the vectors (a⃗ −b⃗ ) and (c⃗ −b⃗ ). (All India 2015)
Answer:

Question 61.
Find the value of λ so that the four points A, B,C and D with position vectors 4 î + 5ĵ + k̂, -ĵ – k̂,3i + Xj+4k and – 4 î + 4ĵ + 4 k̂, respectively are coplanar. (Delhi 2015C)
Answer:
Use the condition that four points with position vectors 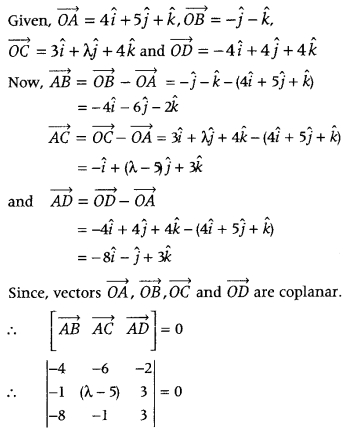A⃗ ,B⃗ ,C⃗  and D⃗  are coplanar, if
[AB−→−,AC−→−,AD−→−]=0→ = 0.

On expanding along R1, we get
⇒ – 4(3λ – 15 + 3) + 6(- 3 + 24) – 2(1 + 8λ – 40) = 0
⇒ – 4(3λ – 12) + 6(21) – 2(8λ – 39) = 0
⇒ – 12λ + 48 + 126 – 16λ + 78 = 0
⇒ – 28λ + 252 = 0
λ = 9

Question 62.
Prove that [a⃗ b⃗ +c⃗ d⃗ ]=[a⃗ b⃗ d⃗ ]+[a⃗ c⃗ d⃗ ]. (All India 2015C)
Answer: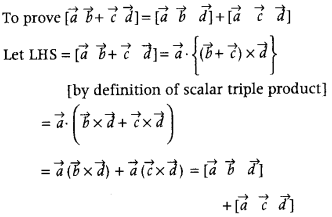

Question 63.
If a⃗  = 2î – 3ĵ + k̂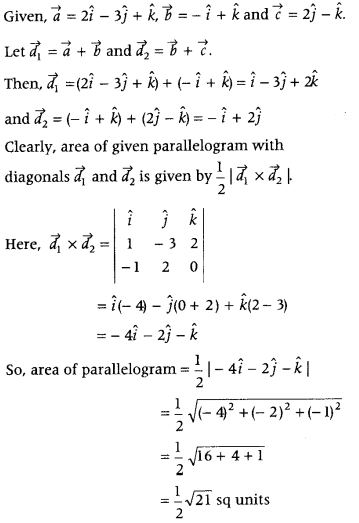, b⃗  = – î + k̂, c⃗  = 2 ĵ – k̂ are three vectors, find the area of the parallelogram having diagonals  and . (Delhi 2014C)
Answer:

Question 64.
Vectors a⃗ ,b⃗  and c⃗  are such that a⃗ +b⃗ +c⃗ =0→ and |a⃗ | = 3, |b⃗ | = 5 and |c⃗ | = 7. Find the angle between a⃗  and b⃗ . (Delhi 2014,2008; All India 2008)
Answer:
π3

Question 65.
The scalar product of the vector a⃗  = î + ĵ + k̂ with a unit vector along the sum of vectors b⃗  = 2î + 4ĵ – 5k̂ and c⃗  = λî + 2ĵ + 3k̂ is equal to one. Find the value of λ and hence, find the unit vector along b⃗  + c⃗ . (All India 2014)
Or
The scalar product of vector i + j + k with the unit vector along the sum of vectors 2î + 4ĵ – 5k̂ and λî + 2ĵ + 3k̂ is equal to one. Find the value of λ. (All India 2009,2008C)
Answer:
First, determine the unit vector of b⃗ +c⃗ , i.e. b⃗ +c⃗ |b⃗ +c⃗ |. Further put a⃗ ⋅(b⃗ +c⃗ )|b⃗ +c⃗ | = 1 and then determine the value of λ.

⇒ (λ + 6)2 = λ2 + 4λ + 44 [squaring both sides]
⇒ λ2 + 36 + 12λ + 4λ + 44
⇒ 8λ = 8
⇒ λ = 1
Hence, the value of λ is 1.
On substituting the value of λ in Eq. (1), we get Unit vector along 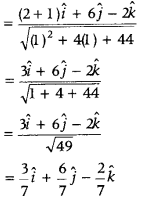b⃗ +c⃗ 

Question 66.
Find the vector p⃗  which is perpendicular to both α⃗  = 4î + 5ĵ – k̂ and β⃗  = î – 4ĵ + 5k̂ and p⃗ . q⃗  = 21, where q⃗  = 3i + j – k. (All India 2014C)
Answer: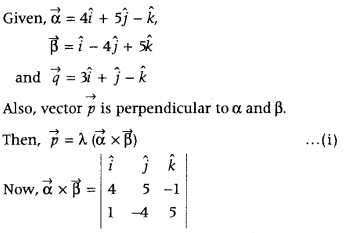

= î (25 – 4) – ĵ (20 + 1) + k̂(- 16 – 5)
= î(21) – ĵ(21) + k̂(- 21)
= 21î – 21ĵ – 21k̂
So, p⃗  = 21λk̂—21λ?—21λk [fromEq.(i)] ….. (ii)
Also, given that p⃗ ⋅q⃗  = 21
∴ (21λî – 21λĵ – 21λk̂) . (3î + ĵ – k̂) = 21
⇒ 63λ – 21λ + 21λ = 21
⇒ 63λ = 21
⇒ λ = 13
On putting λ = 13 in Eq. (ii), we get

which is the required vector.

Question 67.
Find the unit vector perpendicular to both of the vectors a⃗ +b⃗  and a⃗ −b⃗  where, a⃗  = î + ĵ + k̂ and b⃗  = î + 2ĵ + 3k̂. (Foreign 2014)
Answer:

⇒ 2x + 3y + 4z = 0 …… (ii)
and (xî + yĵ + zk̂) . (- ĵ – 2k̂) = 0
⇒ – y – 2z = 0
⇒ y = – 2z
On putting the value of yin Eq. (ii), we get
2x + 3 (- 2z) + 4z = 0
⇒ x = z
On substituting the value of x and y in Eq. (1),
we get
⇒ z2 + 4z2 + z2 = 1
⇒ 6z2 = 1
⇒ z = ± 16√
then, x = ± 16√
and y = ± 26√
Hence, the required vectors are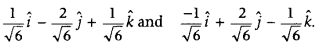

Question 68.
Find the unit vector perpendicular to the plane ABC where the position vectors of A, B and C are 2î – ĵ + k̂, î + ĵ + 2k̂ and 2î + 3k̂, respectively. (All India 2014C)
Answer:
A unit vector perpendicular to plane ABC is
AB−→−×AC−→−|AB−→−×AC−→−|

Let O be the origin of reference.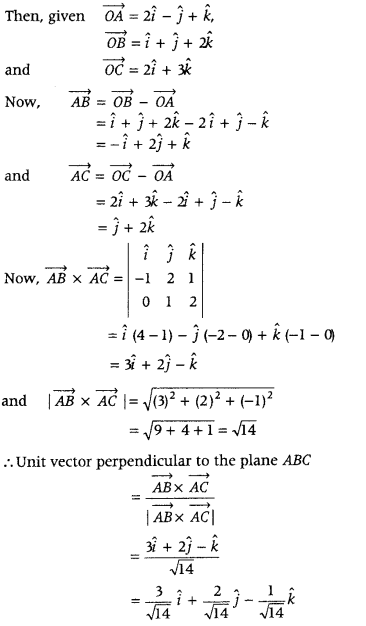

Question 69.
Dot product of a vector with vectors î – ĵ + k̂, 2î + ĵ – 3k̂ and î + ĵ + k̂ are respectively 4, 0 and 2. Find the vector. (Delhi 2013C)
Answer:

⇒ a1 + a2 + a3 = 2
On subtracting Eq. (iii) from Eq. (i), we get
– 2a2 = 2
⇒ a2 = – 1
On substituting a2 = – 1 in Eq. (ii) and (iii),
we get
2a2 – 3a3 = 1 …… (iv)
⇒ a1 + a3 = 3
On multiplying Eq. (v) by 3 and then adding with Eq. (iv), we get
5a1 = 1 + 9 = 10
⇒ a1 = 2
On substituting a1 = 2 in Eq. (v), we get
a3 = 1
Hence, the vector is a⃗  = 2î – ĵ + k̂

Question 70.
Find the values of λ for which the angle between the vectors a⃗  = 2λ2î + 4λĵ + k̂ and b⃗  = 7î – 2ĵ + λk̂ is obtuse. (All India 2013C)
Answer:
let θ be the obtuse angle between the vectors

14λ2 – 7λ < 0
= 2λ2 – λ < 0
Either λ < 0, 2λ – 1 > 0 or λ > 0, 2λ – 1 < 0
= Either λ < 0, λ > 12 or λ > 0, λ < 12 Clearly, first option is impossible. ∴ λ > 0, λ < 12
0 < λ < 12
λ ∈ (0,12)

Question 71.
If a, b and c are three vectors such that each one is perpendicular to the vector obtained by sum of the other two and |a⃗ | = 3, |b⃗ | = 4 and |c⃗ | = 5, then prove that |a⃗ +b⃗ +c⃗ | = 5√2. (All India 2013C, 2010C)
Or
If a⃗ ,b⃗  and c⃗  are three vectors, such that |a⃗ | = 3, |b⃗ | = 4 and |c⃗ | = 5 and each one of these is perpendicular to the sum of other two, then find |a⃗ +b⃗ +c⃗ |. (All India 2011C, 2010C)
Answer:

Question 72.
If a⃗  = 3î – ĵ and b⃗  = 2î + ĵ – 3k̂, then express b⃗  in the form b⃗ =b⃗ 1+b⃗ 2, where b⃗ 1∥a⃗  and b⃗ 2⊥a⃗ . (All India 2013C)
Answer:
Given a⃗  = 3î – ĵ and b⃗  = 2î + ĵ – 3k̂
Let b1→ = x1î + y1ĵ + z1k̂ are two vectors such that b1→+b2→=b⃗ ,b1→∥a⃗  and b2→⊥a⃗ 

Consider, b⃗ 1+b⃗ 2=b⃗ 
⇒ (x1 + x2)î + (y1 + y2)ĵ + (z1 + z2)k̂ = 2î + ĵ – 3k̂

On comparing the coefficient of î ĵ and k̂ both sides, we get
x1 + x2 = 2
y1 + y2 = 1
z1 + z2 = -3

Now, consider b1→∥a⃗ 
⇒ x13=y1−1=z10
⇒ x1 = 3λ, y1 = -λ,and z1 = 0 …(iv)

On substituting the values of x, y and z, from Eq. (iv) to Eq. (i), (ii) and (iii), respectively, we get
x2 = 2- 3λ, y2 = -1 + λ and z2 = -3 …(v)
Since, b2 ± a , therefore b2 a = 0
⇒ 3x2 – y2 = 0
⇒ 3 (2 – 3λ) – (1 + λ) = 0
⇒ 6 – 9λ – 1 – λ = 0
⇒ 5 – 10λ = 0
⇒ λ = 12

On substituting λ = 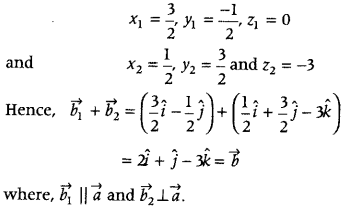12 in Eqs. (iv) and (v), we get

Question 73.
If a⃗  = î + ĵ + k̂ and b⃗  = ĵ – k̂, then find a vector c⃗ , such that a⃗ ×c⃗ =b⃗  and a⃗ ⋅c⃗  = 3. (Delhi 2013, 2008)
Answer:
Given a⃗  = î + ĵ + k̂ and b⃗  = ĵ – k̂
Let c⃗  = xî + yĵ + zk̂

= î (z – y) – ĵ(z – x) + k̂(y – x)
Now, a⃗ ×c⃗ =b⃗  [given]
= î(z – y) + ĵ(x – z) + k̂(y – x)
= 0î + 1ĵ + (-1)k̂ [∵ b⃗  = ĵ – k̂]

On comparing the coefficients from both sides, we get
z – y = 0,x – z = 1, y – x = -1
⇒ y = z and x – y = 1…(i)

Also given, a⃗ ⋅c⃗  = 3
⇒ (î + ĵ + k̂) . (xî + yĵ + zk̂) = 3
⇒ x + y + z = 3 (1)
⇒ x + 2y = 3 [∵ y = z] …(ii)

On subtracting Eq. (i) from Eq. (ii), we get
3y = 2
⇒ y = 23 = z [∵ y = z]

From Eq. (i),
x = 1 + y + 1 = 1 + 23=53
Hence, c⃗ =53i^+23j^+23k^

Question 74.
If a⃗  = î – ĵ + 7k̂ and b⃗  = 5î – ĵ + λk̂, then find the value of λ, so that  and  are perpendicular vectors. (All India 2013)
Answer:
Use the result that if a⃗  and b⃗  are perpendicular, then their dot product should be zero and simplify it.
Given, a⃗  = î – ĵ + 7k̂ and b⃗  = 5î – ĵ + λk̂
Then, a⃗ +b⃗  = (î – ĵ + 7k̂) + (5î – ĵ + λk̂)
= 6î – 2ĵ + (7 + λ) k̂
and a – = (î – ĵ + 7k̂) – (5î – j ̂+ λk̂)
= -4î + (7 – λ)k̂

Since, (a⃗ +b⃗ ) and (a⃗ −b⃗ ) are perpendicular
vectors, then (a⃗ +b⃗ )⋅(a⃗ −b⃗ ) = 0
⇒ [6î – 2ĵ + (7 + λ)k̂]- [-4î + (7 – λ)k̂] = 0 (1)
⇒ -24 + (7+ X)(7 – X) =0
⇒ 49 – λ2 = 24
⇒ λ2 = 25
∴ λ = ± 5

Question 75.
If p = 5î + λĵ – 3k̂ and q = î + 3ĵ – 5k̂, then find the value of λ, so that p⃗ +q⃗  and p⃗ −q⃗  are perpendicular vectors. (All India 2013)
Answer:
λ = ± 1

Question 76.
If a⃗ ,b⃗  and c⃗  are three vectors, such that |a⃗ | = 5, |b⃗ | = 12, |c⃗ | = 13 and a⃗ +b⃗ +c⃗  = 0, then find the value of a⃗ ⋅b⃗ +b⃗ ⋅c⃗ +c⃗ ⋅a⃗ . (Delhi 2012)
Answer:
-169

Question 77.
Let a⃗  = î + 4ĵ + 2k̂, b⃗  = 3î – 2ĵ + 7k̂ and c⃗  = 2î – ĵ + 4k̂. Find a vector p⃗ , which is perpendicular to both a⃗  and b⃗  and p⃗ .c⃗  = 18. (All India 2012,2010)
Answer:
Given vectors are a⃗  = î + 4ĵ + 2k̂,
b⃗  = 3î – 2ĵ + 7k̂
and c⃗  = 2î – ĵ + 4k̂

Let p⃗  = xî + yĵ + zk̂
We have, p⃗  is perpendicular to both a⃗  and b⃗ .
p⃗ ⋅a⃗  = 0
⇒ (xî + yĵ + zk̂) – (î + 4ĵ + 7k̂) = 0
⇒ x + 4y + 2z = 0 ………….(i)

and p⃗ ⋅b⃗  = 0
⇒ (xî + yĵ + zk̂) . (3î – 2ĵ + 7k̂) = 0
⇒ 3x – 2y + 7z = 0 …(ii)

Also, given ~p-~c =18 (1)
⇒ (xî + yĵ + zk̂) . (2î – ĵ + 4k̂) = 0
⇒ 2x – y + 4z = 18 …(iii)

On multiplying Eq. (i) by 3 and subtracting it from Eq. (ii), we get
– 14y + z = 0 ..(iv)

Now, multiplying Eq. (i) by 2 and subtracting it from Eq. (iii), we get
– 9y = 18
⇒ y = -2

On putting y = -2 in Eq. (iv), we get
-14 (-2) + z = 0
⇒ 28 + z = 0
⇒ z = -28

On putting y = -2 and z = -28 in Eq. (i), we get
x + 4 (-2) + 2 (-28) = 0
⇒ x – 8 – 56 = 0
⇒ x = 64

Hence, the required vector is
p⃗  = xî + yĵ + zk̂
i.e. p⃗  = 64î – 2ĵ – 28k̂

Question 78.
Find a unit vector perpendicular to each of the vectors a⃗ +b⃗  and a⃗ −b⃗ , where a = 3î + 2 ĵ + 2k̂ and b = î + 2ĵ – 2k̂. (Delhi 2011)
Answer:
23i^−23j^−13k^

Question 79.
If a and 6 are two vectors, such that |a⃗ | = 2, |b⃗ | = 1 and a⃗ .b⃗  = 1, then find (3a⃗ −5b⃗ )⋅(2a⃗ +7b⃗ ). (Delhi 2011)
Answer: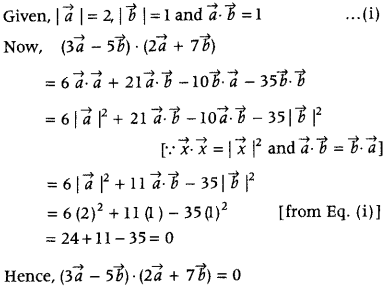

Question 80.
If vectors a⃗  = 2î + 2ĵ + 3k̂, b⃗  = -î + 2ĵ + k̂ and c⃗  = 3î + ĵ are such that a⃗ +λb⃗  is perpendicular to c⃗ , then find the value λ. (Foreign 2011; All India 2009C)
Answer:
Given, a⃗  = 2î + 2ĵ + 3k̂,
b⃗  = -î + 2ĵ + k̂
and c⃗  = 3î + ĵ

Also, a⃗  + λb⃗  is perpendicular to c⃗ .
∴ (a⃗  + λb⃗ ).c⃗  = 0 …(i) [∵ when [latex][/latex], then a⃗ ⋅b⃗  = 0]
Now, a⃗  + λb⃗  = (2î + 2ĵ + 3k̂) + λ (-î + 2ĵ + k̂)
⇒ a⃗  + λb⃗  = î(2 – λ) + ĵ(2 + 2λ) + k̂(3 + λ)
Then, from Bq. (i), we get
[î (2 – λ) + ĵ (2 + 2λ) + k̂(3 + λ)].[3î + ĵ] = 0
⇒ 3(2 – λ) + 1(2+ 2k) = 0
⇒ 8 – λ = 0
∴ λ = 8

Question 81.
Using vectors, find the area of triangle with vertices A (1, 1, 2), 5(2, 3, 5) and C (1, 5, 5). (All India 2011)
Answer:
1261−−√ sq.units

Question 82.
Using vectors, find the area of triangle with vertices A (2, 3, 5), B (3, 5, 8) and C(2, 7, 8). (Delhi 2010C)
Answer:
1261−−√ sq.units